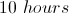Equations
Help Questions
Algebra II › Equations
Solve for 
Explanation


Solve for 
Explanation
To solve for the variable in the linear equation, isolate the variable on one side of the equation with all other constants on the other side. To accomplish this, perform opposite operations when manipulating the equation.
First, add 20x to both sides.

Next subtract 2 from both sides.

Divide both sides by 32.
Solve for 
Explanation
Solve for 
Explanation


Find the solution to the following equation.
Explanation
Find the solution to the following equation.
To find the solution of this equation, we need to find the value for x, which makes the given equation true.
We do this by working backwards and manipulating the equation.
The easiest first step would probably be to subtract 23 from both sides:
By doing so, we can simplify the equation to get:
Next, simply divide both sides by 9 to get the answer!
So we get:
Solve for 
Explanation
In order to solve this equation, we need to isolate the variable, 
Subtract 
Solve.
The answer is not present
Explanation
Isolate the term with x:
Simplify:
Isolate x entirely:
Tom is painting a fence 




If Huck completes painting the entire fence after Tom leaves, how many more hours will Huck work than Tom?
Explanation
Tom paints for a total of 



to determine the total length of the fence Tom paints.

Subtracting this from the total length of the fence 




If Huck works 


Solve:
Explanation
Solve the left side by distribution.
The equation becomes:
Add 
Subtract 2 from both sides.
Divide by 63 on both sides.
The answer is:
Solve the equation:
Explanation
Multiply and distribute the negative five through the binomial.
Subtract 45 from both sides.
Divide by negative five on both sides.
The answer is: