All College Chemistry Resources
Example Questions
Example Question #93 : College Chemistry
Consider the following redox reaction carried out in a voltaic cell.
If the reduction potential for 






For this question, we're given a redox reaction occurring within a voltaic cell. We're also supplied with the reduction potentials for the elements in the reaction, and are asked to find the standard free energy change as well as the equilibrium constant of the reaction at a given temperature.
Recall that in a voltaic cell, the reaction does not consume energy but rather produces it. The anode is where oxidation occurs, while the cathode is where reduction takes place. Based on the reaction given to us in the question stem, the anode will consist of solid 
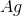




Knowing this, we can calculate 
Now that we have 
A couple things to note. The value for 

To find the value of 

Using 
Using 
As shown above, either method leads us to the same value for 
Certified Tutor
All College Chemistry Resources