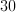Triangles

Help Questions

PSAT Math › Triangles

Questions 1 - 10
1

What is the area of a square that has a diagonal whose endpoints in the coordinate plane are located at (-8, 6) and (2, -4)?

100

100√2

50√2

50

200√2

Explanation

Square_part1

Square_part2

Square_part3

2

What is the hypotenuse of a right triangle with side lengths and ?

Explanation

The Pythagorean Theorem states that . This question gives us the values of and , and asks us to solve for .

Take and and plug them into the equation as and :

Now we can start solving for :

The length of the hypotenuse is .

3

A right triangle has legs of 15m and 20m. What is the length of the hypotenuse?

30m

45m

35m

40m

25m

Explanation

The Pythagorean theorem is a2 + b2 = c2, where a and b are legs of the right triangle, and c is the hypotenuse.

(15)2 + (20)2 = c2 so c2 = 625. Take the square root to get c = 25m

4

In order to get to work, Jeff leaves home and drives 4 miles due north, then 3 miles due east, followed by 6 miles due north and, finally, 7 miles due east. What is the straight line distance from Jeff’s work to his home?

2√5

11

10√2

15

6√2

Explanation

Jeff drives a total of 10 miles north and 10 miles east. Using the Pythagorean theorem (a2+b2=c2), the direct route from Jeff’s home to his work can be calculated. 102+102=c2. 200=c2. √200=c. √100Ÿ√2=c. 10√2=c

5

Screen_shot_2013-03-18_at_10.21.29_pm

In the figure above, is a square and is three times the length of . What is the area of ?

Explanation

Assigning the length of ED the value of x, the value of AE will be 3_x_. That makes the entire side AD equal to 4_x_. Since the figure is a square, all four sides will be equal to 4_x_. Also, since the figure is a square, then angle A of triangle ABE is a right angle. That gives triangle ABE sides of 3_x_, 4_x_ and 10. Using the Pythagorean theorem:

(3_x_)2 + (4_x_)2 = 102

9_x_2 + 16_x_2 = 100

25_x_2 = 100

_x_2 = 4

x = 2

With x = 2, each side of the square is 4_x_, or 8. The area of a square is length times width. In this case, that's 8 * 8, which is 64.

6

The base angle of an isosceles triangle is 15 less than three times the vertex angle. What is the vertex angle?

Explanation

Every triangle contains 180 degrees. An isosceles triangle has one vertex angle and two congruent base angles.

Let = vertex angle and = base angle

So the equation to solve becomes .

7

All of the following could be the possible side lengths of a triangle EXCEPT:

Explanation

The length of the third side of a triangle must always be between (but not equal to) the sum and the difference of the other two sides.

For instance, take the example of 2, 6, and 7.

and . Therefore, the third side length must be greater than 4 and less than 8. Because 7 is greater than 4 and less than 8, it is possible for these to be the side lengths of a triangle.

The 5, 7, 12 answer choice is the only option for which this is not the case.

and . Therefore, the third side length must be between 2 and 12. Because it is equal to the sum, not less than the sum, it is not possible that these could be the side lengths of a triangle.

8

What is the area of a square that has a diagonal whose endpoints in the coordinate plane are located at (-8, 6) and (2, -4)?

100

100√2

50√2

50

200√2

Explanation

Square_part1

Square_part2

Square_part3

9

What is the area of a square that has a diagonal whose endpoints in the coordinate plane are located at (-8, 6) and (2, -4)?

100

100√2

50√2

50

200√2

Explanation

Square_part1

Square_part2

Square_part3

10

What is the hypotenuse of a right triangle with side lengths and ?

Explanation

The Pythagorean Theorem states that . This question gives us the values of and , and asks us to solve for .

Take and and plug them into the equation as and :

Now we can start solving for :

The length of the hypotenuse is .

Page 1 of 45
Return to subject