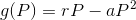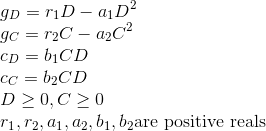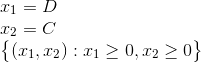All Math Modeling Resources
Example Questions
Example Question #1 : Intro To Dynamic Models
In a meadow there are two types of wild flowers growing, a green floor crawler and yellow daisies. The more desirable flower is the yellow daisy as they can be picked and sold for bouquets. The yellow daisies are also the more slow growing flower of the two. The green floor crawlers grow more rapidly and consume more of the meadow land. Yellow daisies compete with the green flower crawlers by growing taller and shading the crawlers new growth. The yellow daisies are also more resistant to certain types of bugs. Can these two types of flowers coexist on one portion of the meadow, or will one over run the other, driving it to extinction?
Both flower will coexist.
The Green Floor Crawler will go extinct.
Both flowers will thrive.
The Yellow Daisies will go extinct.
The steady state analysis cannot answer the question.
The steady state analysis cannot answer the question.
Since there is competition between the two differing species of flowers, the growth rate function is,
where 

Now identify the question as a dynamic model in steady state.
In other words,
From here, identify what is known about the problem in mathematical terms.
To answer the question determine whether 

To formulate the model let
where the steady state equations are as follows.
For the particular problem, the points on interests are at those where the two functions intersect.
and the intersection of
Using Cramer's rule to solve results in the following.
and when the question of coexistence is ask it is found that,
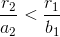
Which after further examination learns to the conclusion that the steady state analysis cannot answer the question.
All Math Modeling Resources